GDP Scientific Goals
Drifter observations have been used in a large number of peer-reviewed scientific studies (see the Drifter Bibliography page). A popular use of the data is to map surface currents and the distribution of eddy kinetic energy. Recent examples (2000 to the present) have focused on the North Atlantic (Flatau et al., 2003), Labrador Sea (Cuny et al., 2002), Caribbean Sea (Centurioni and Niiler, 2003; Richardson, 2004), subtropical eastern Atlantic (Zhou et al., 2000), tropical Atlantic (Grodsky and Carton, 2002; Lumpkin and Garzoli, 2005), eastern South Atlantic (Largier and Boyd, 2001), tropical Pacific (Grodsky and Carton, 2001b; Johnson, 2001; Yaremchuk and Qu, 2004), central subtropical Pacific (Lumpkin and Flament, 2001; Flament et al., 2001), western Pacific (Niiler et al., 2003), North Pacific (Rabinovich and Thomson, 2001), tropical Indian (Grodsky and Carton, 2001a) and Southern (Zhou et al., 2002) oceans. Several recent studies have also focused on marginal seas including the Japan/East (Lee et al., 2000), South China (Centurioni et al., 2004) and Black (Poulain et al., 2004; Zhurbas et al., 2004) seas.
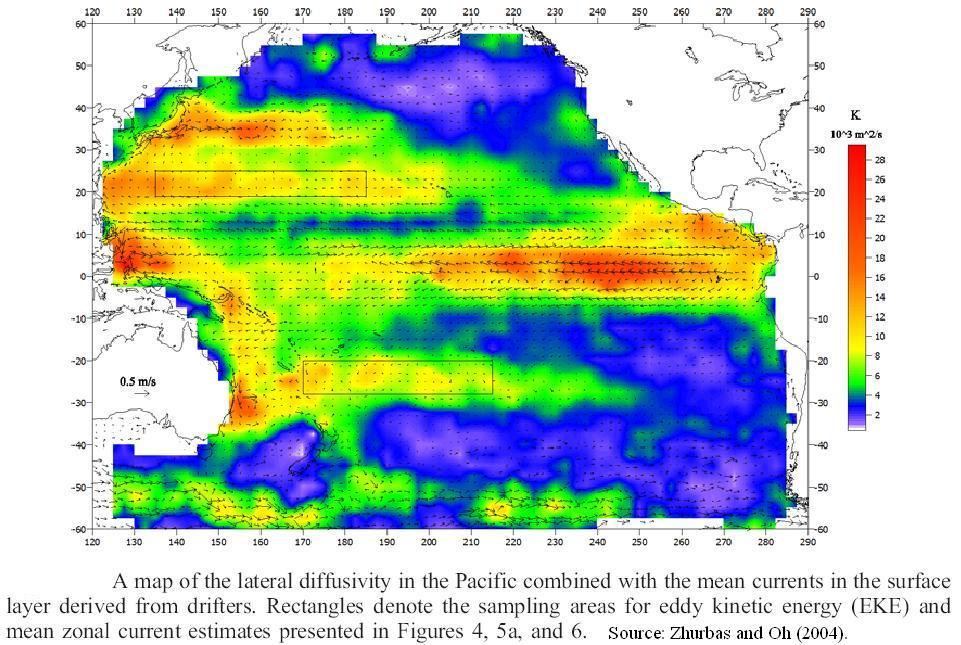
Because drifters follow the two-dimensional surface flow, they are ideal for studying the dispersion of surface particles such as fish larvae and other plankton and buoyant pollutants such as oil spills. Their observations of dispersion can also be used to quantify the effect of mesoscale variability upon mean transports (c.f. Davis, 1991). Early studies of oceanic dispersion from drifter observations (Richardson, 1983; Davis, 1985; Krauss and Boning, 1987) addressed the relevance of Taylor's (1921) classical dispersion theory. Taylor demonstrated that the mean square distance that a particle will diffuse can be characterized in a homogeneous, stationary field of eddies by time and length scales known as the Lagrangian integral scales. These are in principle different from their Eulerian counterparts, although some mixing length-based studies have mapped eddy diffusivities using observations of Eulerian length scales (e.g., Stammer, 1998). Lumpkin et al. (2002) compared the Lagrangian and Eulerian length scales from surface drifters and altimetry, and found that they were proportional only in the most energetic parts of the ocean (such as the Gulf Stream) where Lagrangian particles are advected around eddies at time scales much shorter than the Eulerian time scale. In the tropical Pacific, Bauer et al. (2002) separated mean and eddy drifter velocities using optimized bicubic splines and found that eddy diffusivity in this region is strongly anisotropic: zonal diffusion is up to seven times larger than meridional diffusion, due to parcel trapping in coherent features such as equatorial and tropical instability waves (Bauer et al., 2002). More recently, Zhurbas and Oh (2004) mapped the global surface diffusivity using a methodology designed to avoid contaminating eddy statistics by time-mean flow shear. Their maps of apparent diffusivity revealed enhanced values in zonal bands at 30 in both southern and northern hemispheres. They attributed this to meandering, eddy-rich eastward currents in the North Atlantic (Azores Current) and North Pacific (North Subtropical Countercurrent), and to the westward drift of Agulhas retroflection eddies in the South Atlantic. In the South Pacific, they argued that the distribution indicated the presence of a South Subtropical Countercurrent.
Eddy statistics from SVP drifter observations can be compared to those of simulated drifters in general circulation models, in order to assess how well the eddy-resolving models simulate near-surface turbulence and associated Lagrangian scales. This type of analysis has shown that the 1/12 degree-resolution Miami Isopycnic Coordinate Ocean Model (MICOM) underestimates EKE in the Gulf Stream extension and ocean interior, perhaps due to the climatological forcing employed or the lack of vertical shear in the bulk mixed layer (Garraffo et al., 2001). Similarly, the 1/10 degree-resolution Parallel Ocean Program (POP) model does not reproduce the observed Lagrangian scales, due to the model being too hydrodynamically stable in the surface layer (McClean et al., 2002).
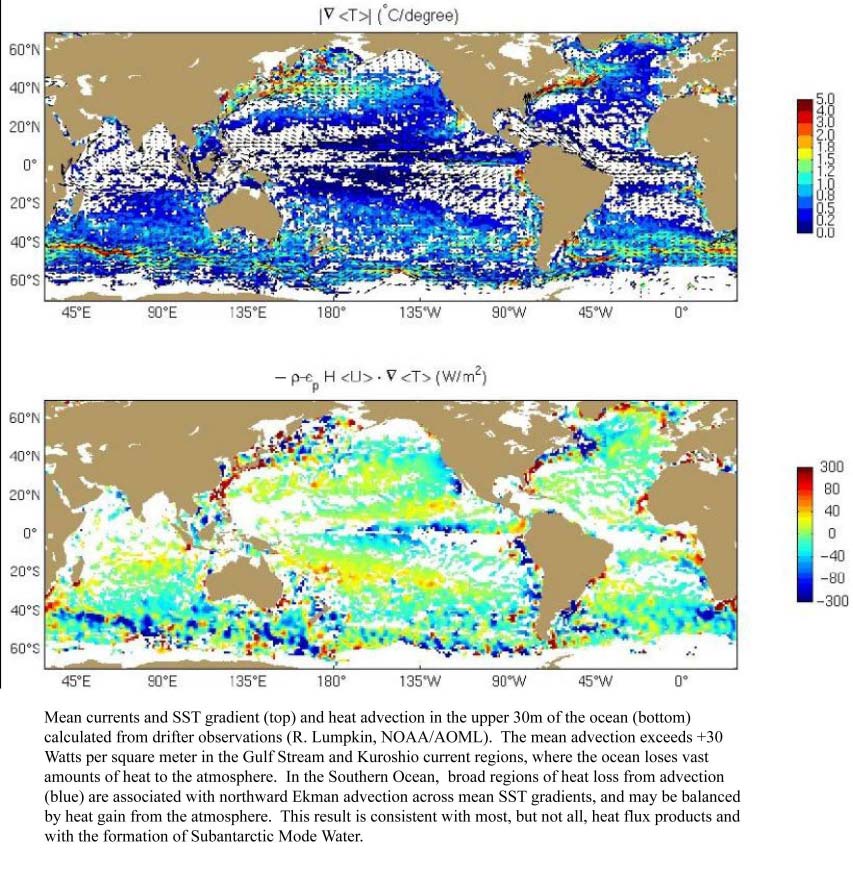
Drifter observations of current and SST are also extremely valuable when evaluating the role of oceanic processes in SST variations. These data have been used to show that tropical instability waves, zonal advection, storage and entrainment all play a major role in the heat budget of the Pacific (Hansen and Paul, 1987; Swenson and Hansen, 1999) and Atlantic (Foltz et al., 2003) equatorial cold tongues. In a recent example, Niiler et al. (2004) have shown that the mean meridional advection of equatorial SST anomalies governs the growth of SST anomalies off the equator in the Nino-3 region during ENSO events.
Many oceanic regions have been sampled very heavily in some times of the year and lightly at other times, due to infrequent batch deployments from research vessels or ships of opportunity. In regions with strong seasonal fluctuations, such as the tropical Atlantic, this will produce biased estimates of the mean flow when the data are averaged in bins. Lumpkin (2003) addressed this problem by dividing the Lagrangian time series into spatial bins and, within each bin, decomposing the time series into time-mean, annual and semiannual harmonics, and a residual. This decomposition was performed using a Gauss- Markov technique familiar in the oceanographic literature for its use in box inverse models (c.f., Ganachaud and Wunsch, 2000). This approach can resolve the amplitudes and phases of seasonal fluctuations throughout most of the tropical Atlantic basin with the present density of SVP drifter observations (Lumpkin and Garraffo, 2005).
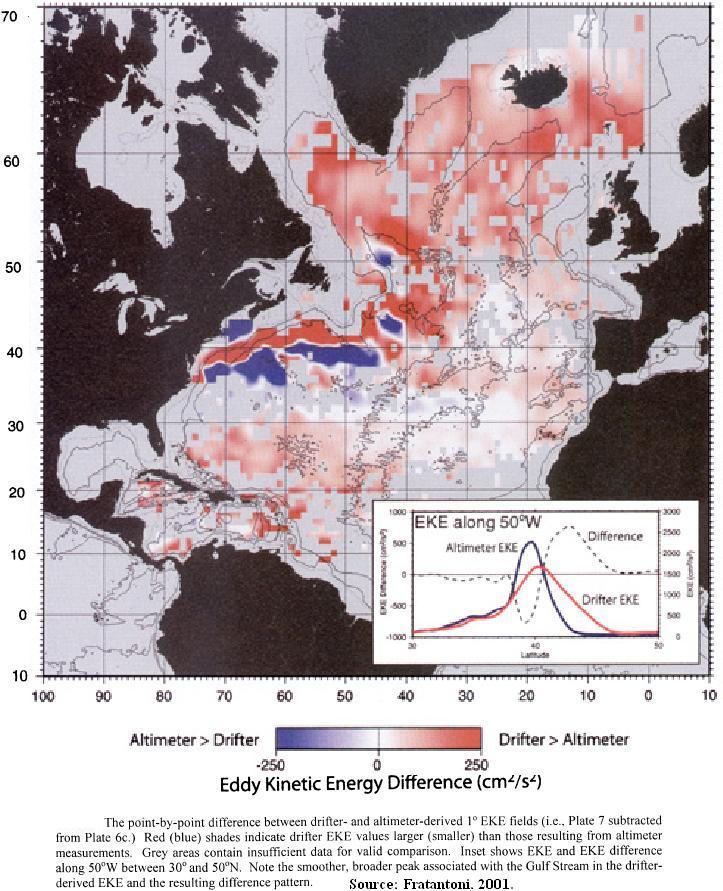
An extremely exciting recent development is the synthesis of SVP drifter observations and satellite altimetry. Altimetry is excellent at capturing the variations of sea level height associated with geostrophic velocity anomalies, but cannot yet map absolute sea level with sufficient accuracy to resolve time-mean currents. Furthermore, sea level anomaly fails to account for significant ageostrophic components of velocity variation, such as centrifugal effects that may account for differences in drifter-derived and altimeter-derived eddy kinetic energy (EKE) on either side of the Gulf Stream front (Fratantoni, 2001).
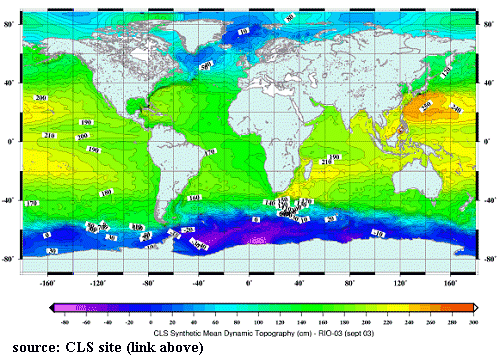
In addition, gridded altimetric products smooth the observations and thus tend to systematically underestimate EKE. Niiler et al. (2003) describe a methodology to synthesize Ekman-removed drifter speeds with gridded altimetric velocity anomalies in regions where they are significantly correlated. This methodology uses concurrent drifter and altimetry velocities in order to calibrate the altimetry, making its amplitude consistent with the in-situ observations, and uses the continuous time series from altimetry to correct for biased drifter sampling of mesoscale to interannual variations. By applying this approach to the global data set of drifter observations, Niiler et al. (2004a) have produced a map of the absolute sea level of the global ocean for the period 1992 2002. In a similar study, Rio and Hernandez (2004) synthesized a geoid model, operational winds, and observations from drifters, altimetry, and hydrography to produce an alternative global mean dynamic topography.
Future work will improve upon these efforts by using results from the Gravity Recovery and Climate Experiment and the rapidly growing data sets of Argo float profiles and drifter trajectories in remote regions such as the Southern Ocean.